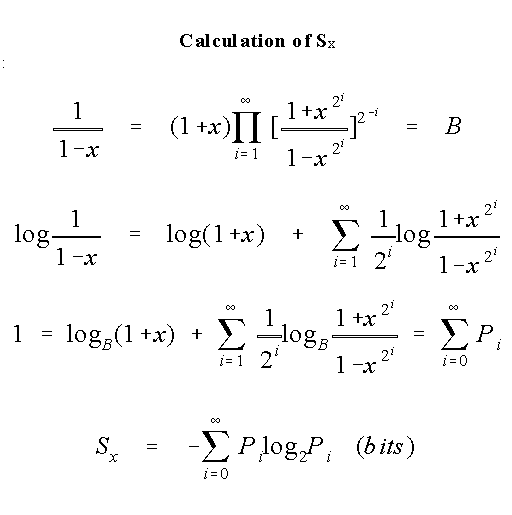Welcome › Forums › Gravitation › Precession of the perihelion of Mercury
- This topic has 32 replies, 2 voices, and was last updated 9 years ago by Gyula Szász.
-
AuthorPosts
-
March 3, 2016 at 5:46 pm #381Gyula SzászModerator
Dear Bill,
the “inverse-square-law” is identical for elementary electric qi and gravitational charges gi up to the overall sign
E(Coulomb)(r) = F (Coulomb)(r) /q = + qi r /4π r³ = +(± e) r /4π r³,
E(Newton)(r) = F(Newton)(r)/g = – gi r /4π r³ = – (± g∙mi) r /4π r³.
From the forces F (Coulomb)(r), F(Newton)(r) the static fields E(Coulomb)(r), E(Newton)(r) can be derived according unit charges by division with arbitrary charges q and g. These expression are not only a static approximations (the bodies which carry the charges does not move), but it is also an approximation in the sense that the distance vector r can be observed exactly (do not forget, at r = 0 resides the particle with the charges qi and gi). Furthermore, it is assumed that no other field is present as the field of the charges qi, gi. and the propagation of the fields are suppressed.
The division by 4π takes care explicit for the full spherical integration. Otherwise as by the electric charges qi = {± e}, the gravitational charges gi = {± g∙mi }can be expressed with the specific gravitational charge g and with the elementary masses me and mP. And g is the same for all four elementary particles e,p,P,E. Thus the Newtonian universal gravitational constant is G = g2/4π or g = + (G∙4π)1/2.
Finally, the values of the charges are the constant values of the full spherical integration, no matter where the charges reside within the sphere, and no matter how large the radius of sphere is (or whatever the closed surface should be).
Thinking on time dependency, the values of elementary charges are constants; they are invariants.
The elementary masses of the elementary particles are also constant under the assumption of equal specific gravitational charge g for all four elementary particles. In this sense, the gravitational masses of bodies are conserved; however, at the calculation of the gravitational masses > 0 of bodies, the signs and numbers of elementary particles must be taken into considerations which build the body.
We did not speak about equation of motion yet. The equation of motion for elementary particles with the elementary gravitational charge gj in the field of another elementary particle (in the approximation described above), regarding only the gravitational force is
mj ∙ a = Fij (Newton)(rij) = – gi∙ gj rij/4π rij³ = – (± g²∙mi∙mj) r/4π r³= – (± G∙mi∙mj) r/r³.
You see, you can cancel the mass mj, but it remains the relative signs of the two gravitational charges. For elementary particles the inertial and gravitational masses have the same values.
For two electric neutral composed bodies with the same sign of the gravitational charges, you would have the equation of motion of a body (in the static approximation) in the gravitational field of a second body, BODY,
mi (body) ∙ a(body) = F (Newton)(rij) = – G∙mg(BODY)∙mg(body) r/r³,
whereby the inertial mass of the body is
mi (body) = Σ Nj ∙ mj – E(body;bound)/c² = (NP-NE) mP+(Np-Ne) me – E(body;bound)/c²,
and the gravitational mass is (supposing there are no eltons present)
mg (body) = N ∙ (mP –me).
Clearly, the inertial masses and gravitational masses for composed bodies are different. Thus, the Newtonian equation of motion must be adjusted because the inertial and gravitational masses are different.
Other changes for the equation of motion arise if the gravitational field is treated as time dependent field with the propagation of c. The first order of change in v/c brings the gravito-magnetic effect. This effect is energy conserved yet, because the velocity v is perpendicular to the gravito-magnetic field B(g)(r) . Energy violations occur if one consider terms in order of O((v/c)²).
You must take these all into consideration if you want treat the fast stars in spiral galaxies!
Sincerely,
GyulaMarch 4, 2016 at 7:46 am #401Gyula SzászModeratorThe Atomistic Theory of Matter and the Theory of Gravitation belong scientifically together. The gravitation is caused by conserved elementary gravitational charges. As a result of these circumstances is that the deformation of space-time about masses, as the general relativity stated, in not a physical correct description of gravitation.
The Standard Model of Astrophysics speculate scientifically about Big Bang, Black Holes, Dark Matter, deviation of light in the gravitational field, event horizon, accelerated expansion of the Universe, etc. However, all these scientific speculations have no physical basis.
The detection of gravitation waves – published by Abbott et al., Phys. Rev. Lett, 11. February 2016 – was firstly successful. However, the origin of the gravitation waves was not the merger of two Black Holes, but rather the merger of two neutron stars. “Cold” neutron stars have the greatest mass density in Universe, ca. 3.8 x10^12 kg/cm^2.
Not theories have to say what Nature is, but Nature requires the description with consistent and comprehensive theories.
March 4, 2016 at 9:45 am #403Gyula SzászModeratorI have worked out the “Lorentz-Invariant Theory of Gravitation” on the basis of “Fundamental Principles in Physics” and will be published soon. The fundamental principles lead to Atomistic Theory of Matter. The theory has unified the gravitation with the electromagnetism.
March 5, 2016 at 11:29 am #406Gyula SzászModeratorDear Gyula,
This was to be my first post on your Gravitations forum:
The subject would be COVARIANCE
I’d like to discuss covariance. I have attached
a picture of the identity of 1/(1-x), what I will
call the “implicit covariant factor.” The right
hand side of the identity I will call the “explicit
covariant factor.” Never mind, for now, how the
probabilities and Shannon Entropy packets are
derived, they only introduce concepts that the
reader may not be familiar with, yet. It is left
as an exercise to show that 1/(1-x) has as a second
family member, [1/(1-x^2)]^0.5, the covariant Lorentz
factor when (v/c) is substituted for x, where the
first factor is the Pythagorean way to add vectors.
That is, the whole right hand side is how to add
vectors covariantly; the Lorentz way.Now for the intuition; since the first factor on the
right hand side of the identity is (1+x), then I
suggest that the whole right hand side is how to
add scalars covariantly; the proposed way.So you might ask? “Covariant inertia explains the
correct way to view simultaneous, synchronous, and
coincidental events…what would your so-called
covariant addition give us a correct view of?”Umm, maybe a correct view of where gravity waves
eminate from… maybe from the covariant part,
and conserving the gravitational mass addition as
a simple (conserved) addition with the substitution
of GM/R/(c^2) for x; where the first power of M is
an un-creatable, un-annihilatable, constant of the
universe, the gravitational mass. And the possibility
that the covariant part is a “captive wave,” capable
of being radiated.Intuition or fantasy? Why or why-not? Don’t ask me,
I just like the thought of something like “covariant
addition,” and can not help it. Lorentz and Einstein
taught us why covariance is necessary to fix the
Lorentz transform matrix by multiplying by the Lorentz
factor;my feeling is that the addition of gravitational masses
is also covariant. Rule-me-out, but please don’t throw
me onto the brier-patch, said brother-rabbit to brother-
bear.And if that isn’t enough, that the covariance is a first
principle that demands that the speed of light and gravity
be finite and equivalent, a reversal of a “common-sense”
notion of causality; and that covariance is the property
that accounts for chemical electromagnetic bonds on the
inertial side of the equation and nuclear electromagnetic
bonds on the gravitational side of the equation.Sincerely,
Bill (Eshleman)Dear Bill,
the role of mass, as used in physics, is widely discussed in
https://en.wikipedia.org/wiki/Mass_in_special_relativity
One statement was “the expression m0(1 – v2/c2)−1/2 is best suited for THE mass of a moving body”.
It is argued that the relativistic mass formula holds for all particles, including those moving at the speed of light, while the formula only applies to a slower than light particle (a particle with a nonzero rest mass) with ϒ = (1-v^2/c^2)^-1/2.However, the relation E = m(rel)∙c^2 (Einstein) is generally invalid; firstly because moving particles always radiate and secondly, it is not distinguished between the gravitational mass mg and the inertial mass mi. In general, the energy E of moving particle is not conserved and the mg and mi are different by composed particles. Only by the elementary particles, e, p, P and E, we can be sure that mg = mi because they are not composed of other particles. The statement that E = m(rel)∙c^2 holds for all particles is a physically invalid claim.
Supposing all particles are composed of the elementary particles e, p, P and E the is the gravitational mass
mg = |(NP – NE)mP + (Np-Ne)me|
and the inertial mass
mi = (NP + NE) mP + (Np + Ne) me – E(bound)/c^2.
Both masses are greater or equal zero and are expressed with the numbers of elementary particles, with the elementary masses mP and me and in the inertial mass also the bound energy E(bound) enters.
COVARIANCE is connected to the covariant equation of motion of particles, which carrying two kinds of fields, however, neither the positions, nor the velocities of particles are exactly known. This uncertainty is a principle problem at the motion of particles. In multilinear algebra and tensor analysis, covariance and contravariance describe how the quantitative description of certain geometric or physical entities changes with a change of basis. In physics, a basis is sometimes thought of as a set of reference axes. However, as a “set of reference axes” cannot be connected to “inertial frame of references” because the observers cannot define constant velocities with real physical particles. In the Minkowski space a basis with four unit vectors e0, e1, e2 , e3 can be introduced, and also an invariant distance between two points. However, it is dangerous to define covariance with the use of Lorentz factor ϒ = (1-v^2/c^2)^-1/2. In the equation of motion of particles all appearing term are covariant terms.
March 5, 2016 at 1:48 pm #407Gyula SzászModerator“The relativistic expressions for E and p obey the relativistic energy–momentum relation:
E^2 – (pc)^2 =(mc^2)^2
where the m is the rest mass, or the invariant mass for systems, and E is the total energy”
First of all, is the “invariant rest mass” the rest inertial mass or the gravitational mass for the system? These are generally different. And what is the “total energy”? Furthermore, the energy is not conserved. This equation is an illegal oversimplification and has no relevance in physics. I’m wondering why such an equation is used in academic physics. The energy is not connected with the impulse p according this equation with the mass. Furthermore, the conservation of energy is unable to determine the equation of motions of particles. The whole definition of the theory of special relativity (1905, Einstein) is only a catchphrase and is scientifically without value.
If one would describe the four elementary particles, i=e,p,P,E, without radiation field and without interaction to the field, one would have the expression for the Lagrange density
L(particles)(x) = ∑ (i=e,p,P,E ) mi ⋅ c ⋅ ∂ ν ji(n) ν (x)
with ji(n) ν (x) = ( c ρi(n) (x), ji(n) (x) ) the particle number probability density and the particle numbers are conserved. Here are the principle uncertainty for the determination of the positions and velocities of particles contained. The integration about finite regions of Minkowski space Ω gives the action integral for the derivation of the equation of motions of the particles, however the subsidiary condition of particle number conservation must also be fulfilled. What Einstein did 1905 was nothing at the definition of special relativity.(unfortunately the text editor does not transfer the lower and upper indexes)
- This reply was modified 9 years, 1 month ago by Gyula Szász.
March 5, 2016 at 2:03 pm #408Gyula SzászModeratorThe correct written formula for the Lagrange density you can find on this website under Theory in “Atomistic Theory of Matter: Stable Particles and a Unified Field” as Eq. (37).
March 5, 2016 at 7:26 pm #410Gyula SzászModeratorDear Gyula,
Here is the hastily prepared response that I wish
to post to Gravitations:“Ich weiß nicht sicher”, but my identity seems to
separate the “rest mass” (?gravitational mass?)
from its motion part; a hopeful but certainly
uncertain conclusion to draw. But nonetheless
attractive path to investigate.If “covariance” is the wrong concept to attribute
to my infinite product of the ratios of polynomial
conjugates identity, then I am fine with calling it
“The XS” for now. But the “XS” serves to modify
the Pythagorean addition of vectors, so I’m
inclined to speculate that it is “ad hoc”, the
special relativistic part.of the Lorentz factor.Got any suggestions as to what we might call
that XS special relativistic part? It looks
“covariant” to me, but its being certainly
hypothetical, and its being a never before seen
mathematical object, folks who I shown it to
seem to universally suggest that it is a pure
mathematical object having no applicability at
all. When I discussed my interpretation with
“mentors” on the SciAmPhysicsForum, I ended
up being “banned for life”, for teaching crack-
pottery. 🙁Sincerely,
Bill EshlemanMarch 6, 2016 at 8:53 am #411Gyula SzászModeratorDear Bill,
The assumption of energy conservation cannot be applied for physical systems because all physical systems are open (non-closed) systems. Furthermore, all interactions are non-conservative interactions (the particles radiate and lost energy). Due to radiations, neither the positions, nor the velocities of particles are exactly known at a fixed time. Therefore, the particles must be described with probability densities. Since the interactions propagate with c, the Minkowski space has to be used for the description of space-time processes. These features are not built in the fundaments of physics.
What is about conservation laws? The only remaining law is the conservation of particle numbers. The particle number conservations of the elementary particles are connected to charge conservations. The elementary charges are on one side the physical properties of elementary particles, on the other side they cause the non-conservative, continuous interactions which propagate with c.
The experimentally observed stable elementary particles are the electrons (e), the positrons (p), the protons (P) and the eltons (E). I have labeled the “antiproton” as elton. The four kinds of elementary particles carry two kinds of conserved elementary charges, the elementary electric charges qi ={±e} and the elementary gravitational charges gi = {±g ∙mi}. The elementary gravitational charges can be expressed with the elementary masses me and mP and with the specific gravitational charge g whereby the connection between g and the universal gravitations constant G is g = + (G∙4∙π)^1/2. The gravitation is not universal mass attraction and the gravitation is also not caused by the deformation of space-time around masses. However, the Universality of Free Fall (UFF) is violated.
The energetic physics which use the energy conservation and which is developed in the 20th century is not suitable to describe correctly physical processes. Instead of the energetic physics, the atomistic physics, built on four kinds of stable particles, must be used in physics.
Final remark: Not physical theories have to say what Nature is, but Nature requires the correct and comprehensive physical descriptions.
Sincerely,
Gyula- This reply was modified 9 years, 1 month ago by Gyula Szász.
March 7, 2016 at 8:19 am #421Gyula SzászModeratorBill: And please let me add….
“I’m thinking that Einstein used the infinite sum
identity for the Lorentz factor because it’s so
easy to differentiate but places the first power
of the gravitational mass on every term.But not so for infinite product identities for
the Lorentz factor which are difficult to differentiate
but only need the gravitational mass to appear
in its first factor and no others.And I am speculating that it is the influence of the
choice of treatments that accounts for properties
that don’t commute. An ambition which I have not
succeeded at yet.”No, Einstein did not understand what mass is. He threw away the gravitational masses.
The gravitational mass is in the sense invariant that the gravitational charges are invariant.
And the gravitational charges are connected by a factor g =(G4π)^1/2 with the universal gravitational constant G to the gravitational masses.
The Lorentz factor is not a correct factor to describe “relativistic masses”.During one could consider the gravitational mass as “invariant mass” within the above consideration, the “relativistic inertial mass” comes out of the correct equation of particles motion.
Gyula- This reply was modified 9 years, 1 month ago by Gyula Szász.
March 8, 2016 at 7:07 am #423Gyula SzászModeratorDear Gyula,
Thank you for pasting the picture. I am still trying
to recover from your statement that the Lorentz factor
is an incorrect, special relativistic correction for
gravitational and electric charges. The most important
problem with the Lorentz factor is its infinity at the
speed of light. An infinity that is a curtain both to the
hypothetical black hole and to the microscopic quantum
world. Worlds that may just become visible by a
Feynman-type renormalization….maybe.I am hoping that my splitting the Lorentz factor into a form
consisting of a purely additive part,(addition of both ideal scalars and vectors),
and a purely, but speculated “relativistic” part………..will
come to my aid so that we can agree; always with my
primary intent to agree with your theory, “come hell or
high water.” And as of now, the extra factors of my
mathematical objects, I am interpreting as trying to
describe what the energies of bonds must approximate
to achieve longevity and stability. And it is those factors
that contribute to the infinities, whereas the first conservative
factor has no infinity at all.My effort will usually have the goal that we agree in
some way that I can justify by manipulation of the counters
on my infinite sum and product identities. That is, it’s
treatment is flexible or even correctible on my part.
Sincerely,
Bill EshlemanDear Bill,
you must think on the equation of particles motion under the condition that neither the positions, no the velocities of particles are ever exactly known. We can assume that for stable particles the gravitational mass is equal to the inertial mass (they are not composed of any other particles), but the Lorentz factor contain the term v/c and which velocity will you input for v? Furthermore, the interactions between particles are non-conservative interactions. Anyhow, as the equations of motions are derived from an invariant action integral, the validity is given at each velocities smaller than c.
I state once more again the equation of stable particles motion is a covariant equation and it is composed of at least three covariant terms (the interaction term appears two times, the radiation term is suppressed). Covariant means “form invariant” again passive Lorentz transformations, and passive Lorentz transformations mean transformations of the coordinate system. The mass appears in two terms, in the kinetics part and in the interaction part through the gravitational interaction; in both cases the masses of stable particles enter as invariant masses.
If somebody tries to describe composed particles/bodies, in the kinetics part, the so called inertial mass occurs (which is not an invariant) in the interaction term appears the sum of gravitational charges as sum of gravitational masses. The inertial mass incorporates the bound energy. The uncertainty of velocities and the non-conservative interactions remain the same also for composed particles/bodies.
In my microscopic quantum word renormalization a la Feynman is not needed anymore and the particles cannot approach each other infinitely close and they cannot move with the velocity c. Furthermore, black holes do not exist; the space-time is not “deformed”.
Sincerely,- This reply was modified 9 years, 1 month ago by Gyula Szász.
March 8, 2016 at 9:29 pm #425Bill EshlemanParticipantDear Gyula,
Whenever you mention that things about the dynamics
of the universe cannot be exactly known, a little bird
whispers in my ear that we cannot know ANYTHING exactly.
But I suspect at the same “whisper,” that, that is not
exactly what you are implying either.The “whisper” tells me that you are implying that the
“Laws of Nature” are also ambiguous because they don’t
know ANYTHING exactly, either.So I suggest, like times before, that all that can be
known are “averages”; averages that are better approximations, and again never exact, but only a better “fit” with mathematical models. And that the
“Laws of Nature” are limited to never knowing what
things are, but only what they were.Invariance, covariance, form invariance… these are
“buzz-words” to me…..So I suggest that their root cause, or rather their
first principle, are relationships between polynomials
without cross-products and their appropriate conjugates.
Not as a result of, but the cause of invariance, etc.That is, the Lorentz factor and my proposed Gravitational
factor are analytical approximations of the bonding of
atoms into molecules via the motion of electrons, and the
bonding of protons and neutrons onto nuclei via vibrational
motion of charges, both electrical and gravitational.What I see as merely an ambition, other folks might view
as incorrigibility. 🙁Sincerely,
Bill EshlemanMarch 8, 2016 at 10:53 pm #426Bill EshlemanParticipantAnd if that isn’t incorrigible enough for your
sensibilities, that static wave-like energy is
stored in composite particles as what might be
loosely called “The Conjugates of fields”, and
that radiations are due to the orthogonality of
the very same fields; two different forms of the
same thing; one static and the other dynamic.March 8, 2016 at 11:43 pm #427Bill EshlemanParticipantAnd no, please don’t accuse me as trying to
dominate this thread; it’s just that I agree
and intend to agree with the atomistic viewpoint
and am merely speculating on the composition of
the invisible lines-of-force between participating
particles. Lines of force idealized and imagined
as iron particles on a sheet of paper with a magnet
underneath, but otherwise quite invisible.I’m saying this as an experienced coder of numerical
simulations of things, NOT as a physicist.So my image of those invisible lines of force as not
only being described by conjugate polynomials, but also
caused by the conjugate polynomials, might, as some say,
be too good to be true, and therefore most likely false.So I elevate conjugate-ness to a first principle in
retribution….. makes sense to me….March 9, 2016 at 1:44 am #428Bill EshlemanParticipantSo one may ask….”What is the connection between
The Szaszian Atomistic treatment of reality, and
Eshleman’s Entropic treatment?”Ludwig Boltzmann
March 9, 2016 at 7:26 pm #429Gyula SzászModeratorDear Bill,
I can precisely say what the atomistic treatment of “reality” is:
The atomistic treatment describe matter and interacting fields correctly (for instant at each velocities of particles; no matter how large the velocities are) under the circumstance that neither the positions, nor the velocities are ever exactly known. For the description, only invariants are used (for instant invariant elementary charges, invariant terms in the Lagrange density, invariant action integral, etc.) in order to get covariant equations of motions; that means: equations of motions which are independent from coordinate systems (and are valid for all velocities of particles). That is exactly the dynamics of the universe. The laws of nature are non-deterministic, however causal.You said “Invariance, covariance, form invariance… these are “buzz-words” to me…..”. I’m unhappy to hear this. You try to use treatments, either such within you don’t use invariants (entropic treatment), or you tries to use exactly known velocities (Lorentz factor with a mass, but which mass?). If you want, there is little connection between our both theoretical settings.
-
AuthorPosts
- You must be logged in to reply to this topic.